Logarithm (nonfiction): Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| Line 1: | Line 1: | ||
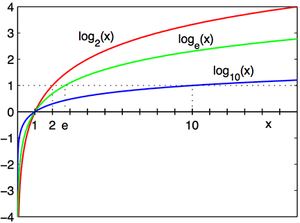
[[File:Logarithm_plots.png|thumb|Plot of logarithm function for three common bases.]]In [[Mathematics (nonfiction)|mathematics]], the '''logarithm''' is the inverse operation to exponentiation, just as division is the inverse of multiplication and vice versa. | [[File:Logarithm_plots.png|thumb|Plot of logarithm function for three common bases.]]In [[Mathematics (nonfiction)|mathematics]], the '''logarithm''' is the inverse operation to [[Exponentiation (nonfiction)|exponentiation]], just as division is the inverse of multiplication and vice versa. | ||
The logarithm of a number is the exponent to which another fixed number, the base, must be raised to produce that number. | The logarithm of a number is the exponent to which another fixed number, the base, must be raised to produce that number. | ||
| Line 18: | Line 18: | ||
== Nonfiction cross-reference == | == Nonfiction cross-reference == | ||
* [[Exponentiation (nonfiction)]] | |||
* [[Super-logarithm (nonfiction)]] | * [[Super-logarithm (nonfiction)]] | ||
* [[Mathematics (nonfiction)]] | * [[Mathematics (nonfiction)]] | ||
Revision as of 10:22, 18 November 2017
In mathematics, the logarithm is the inverse operation to exponentiation, just as division is the inverse of multiplication and vice versa.
The logarithm of a number is the exponent to which another fixed number, the base, must be raised to produce that number.
In simple cases the logarithm counts factors in multiplication. For example, the base 10 logarithm of 1000 is 3, as 10 to the power 3 is 1000 (1000 = 10 × 10 × 10 = 103); 10 is used as a factor three times.
More generally, exponentiation allows any positive real number to be raised to any real power, always producing a positive result, so the logarithm can be calculated for any two positive real numbers b and x where b is not equal to 1.
In the News
Fiction cross-reference
Nonfiction cross-reference
External links:
- Logarithm @ Wikipedia