Hopf fibration (nonfiction)
Jump to navigation
Jump to search
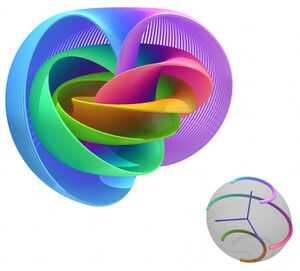
In the mathematical field of topology, the Hopf fibration (also known as the Hopf bundle or Hopf map) describes a 3-sphere (a hypersphere in four-dimensional space) in terms of circles and an ordinary sphere.
Discovered by Heinz Hopf in 1931, it is an influential early example of a fiber bundle. Technically, Hopf found a many-to-one continuous function (or "map") from the 3-sphere onto the 2-sphere such that each distinct point of the 2-sphere comes from a distinct circle of the 3-sphere (Hopf 1931).
Fiction cross-reference
Nonfiction cross-reference
External links
- Hopf fibration @ Wikipedia