Pencil (mathematics) (nonfiction): Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| Line 1: | Line 1: | ||
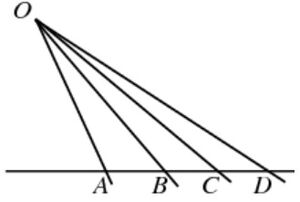
In [[Projective geometry (nonfiction)|projective geometry]], a '''pencil''' is a family of geometric objects with a common property, for example the set of lines that pass through a given point in a projective plane. | [[File:Pencil (mathematics).jpg|thumb|Four lines passing through a given point in a projective plane.]]In [[Projective geometry (nonfiction)|projective geometry]], a '''pencil''' is a family of geometric objects with a common property, for example the set of lines that pass through a given point in a projective plane. | ||
In affine geometry with the reflexive variant of parallelism, a set of parallel lines forms an equivalence class called a pencil of parallel lines. | In affine geometry with the reflexive variant of parallelism, a set of parallel lines forms an equivalence class called a pencil of parallel lines. | ||
Revision as of 19:38, 9 November 2018
In projective geometry, a pencil is a family of geometric objects with a common property, for example the set of lines that pass through a given point in a projective plane.
In affine geometry with the reflexive variant of parallelism, a set of parallel lines forms an equivalence class called a pencil of parallel lines.
More generally, a pencil is the special case of a linear system of divisors in which the parameter space is a projective line.
A pencil of planes, the family of planes through a given straight line, is sometimes referred to as a fan or a sheaf.
The term was first used by Girard Desargues (Cremona 1960, p. x).
Fiction cross-reference
Nonfiction cross-reference
External links:
- Pencil (mathematics) @ Wikipedia
- Pencil @ Wolfram MathWorld