Imaginary unit (nonfiction): Difference between revisions
(Created page with "thumb|''i'' in the complex or cartesian plane. Real numbers lie on the horizontal axis, and imaginary numbers lie on the vertical axis.The '''imagi...") |
No edit summary |
||
| (3 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
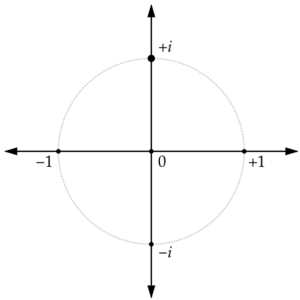
[[File:Imaginary unit.svg|thumb|''i'' in the complex or cartesian plane. Real numbers lie on the horizontal axis, and imaginary numbers lie on the vertical axis.]]The '''imaginary unit''' or '''unit imaginary number''' (''i'') is a solution to the quadratic equation x2 + 1 = 0. There is no [[Real number (nonfiction)|real number]] with this property, hence the term imaginary. | [[File:Imaginary unit.svg|thumb|''i'' in the complex or cartesian plane. Real numbers lie on the horizontal axis, and imaginary numbers lie on the vertical axis.]]The '''imaginary unit''' or '''unit imaginary number''' (''i'') is a solution to the quadratic equation x2 + 1 = 0. There is no [[Real number (nonfiction)|real number]] with this property, hence the term imaginary. | ||
''i'' can be used to extend the real numbers into [[Complex number (nonfiction)|complex numbers]], using addition and multiplication. A simple example of the use of ''i'' in a complex number is 2 + 3''i''. | |||
Imaginary numbers are an important mathematical concept, which extend the real number system ℝ to the complex number system ℂ, which in turn provides at least one root for every nonconstant polynomial P(x). | Imaginary numbers are an important mathematical concept, which extend the real number system ℝ to the complex number system ℂ, which in turn provides at least one root for every nonconstant polynomial ''P(x)''. | ||
There are two complex square roots of −1, namely i and −i, just as there are two complex square roots of every real number other than zero, which has one double square root. | There are two complex square roots of −1, namely i and −i, just as there are two complex square roots of every [[Real number (nonfiction)|real number]] other than zero, which has one double square root. | ||
In contexts where ''i'' is ambiguous or problematic, ''j'' or the Greek ι is sometimes used. In the disciplines of electrical engineering and control systems engineering, the imaginary unit is normally denoted by ''j'' instead of ''i'', because ''i'' is commonly used to denote electric current. | In contexts where ''i'' is ambiguous or problematic, ''j'' or the Greek ι is sometimes used. In the disciplines of electrical engineering and control systems engineering, the imaginary unit is normally denoted by ''j'' instead of ''i'', because ''i'' is commonly used to denote electric current. | ||
| Line 12: | Line 12: | ||
<gallery> | <gallery> | ||
File:Complex number Argand diagram.svg|link=Complex number (nonfiction)|A [[Complex number (nonfiction)|complex number]] can be visually represented as a pair of numbers (a, b) forming a vector on a diagram called an Argand diagram, representing the complex plane. "Re" is the real axis, "Im" is the imaginary axis, and i satisfies i2 = −1. | |||
</gallery> | </gallery> | ||
| Line 19: | Line 20: | ||
* [[Gnomon algorithm]] | * [[Gnomon algorithm]] | ||
* [[Gnomon Chronicles]] | * [[Gnomon Chronicles]] | ||
* [[Mathematics]] | * [[Mathematics]] | ||
== Nonfiction cross-reference == | == Nonfiction cross-reference == | ||
* [[Complex number (nonfiction)]] | |||
* [[Imaginary number (nonfiction)]] - a [[Complex number (nonfiction)|complex number]] that can be written as a [[Real number (nonfiction)|real number]] multiplied by the imaginary unit i,[note 1] which is defined by its property i2 = −1. | |||
* [[Mathematics (nonfiction)]] | * [[Mathematics (nonfiction)]] | ||
* [[Real number (nonfiction)]] | |||
External links: | External links: | ||
Latest revision as of 14:13, 19 February 2019
The imaginary unit or unit imaginary number (i) is a solution to the quadratic equation x2 + 1 = 0. There is no real number with this property, hence the term imaginary.
i can be used to extend the real numbers into complex numbers, using addition and multiplication. A simple example of the use of i in a complex number is 2 + 3i.
Imaginary numbers are an important mathematical concept, which extend the real number system ℝ to the complex number system ℂ, which in turn provides at least one root for every nonconstant polynomial P(x).
There are two complex square roots of −1, namely i and −i, just as there are two complex square roots of every real number other than zero, which has one double square root.
In contexts where i is ambiguous or problematic, j or the Greek ι is sometimes used. In the disciplines of electrical engineering and control systems engineering, the imaginary unit is normally denoted by j instead of i, because i is commonly used to denote electric current.
In the News
A complex number can be visually represented as a pair of numbers (a, b) forming a vector on a diagram called an Argand diagram, representing the complex plane. "Re" is the real axis, "Im" is the imaginary axis, and i satisfies i2 = −1.
Fiction cross-reference
Nonfiction cross-reference
- Complex number (nonfiction)
- Imaginary number (nonfiction) - a complex number that can be written as a real number multiplied by the imaginary unit i,[note 1] which is defined by its property i2 = −1.
- Mathematics (nonfiction)
- Real number (nonfiction)
External links:
- Imaginary unit @ Wikipedia