Analytic geometry (nonfiction): Difference between revisions
(Created page with "In classical mathematics, '''analytic geometry''', also known as '''coordinate geometry''' or '''Cartesian geometry''', is the study of geometry usin...") |
No edit summary |
||
| (4 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
In classical mathematics, '''analytic geometry''', also known as '''coordinate geometry''' or '''Cartesian geometry''', is the study of [[Geometry (nonfiction)|geometry]] using a coordinate system. This contrasts with synthetic geometry. | [[File:Cartesian coordinate system.svg|thumb|Diagram of a [[Cartesian coordinate system (nonfiction)|Cartesian coordinate system]] showing the [[Origin (nonfiction)|origin]], [[Axis (nonfiction)|axes]], and the four [[Quadrant (nonfiction)|quadrants]], with illustrative [[Point (nonfiction)|points]] and grid.]]In classical mathematics, '''analytic geometry''', also known as '''coordinate geometry''' or '''Cartesian geometry''', is the study of [[Geometry (nonfiction)|geometry]] using a [[Coordinate system (nonfiction)|coordinate system]]. This contrasts with [[Synthetic geometry (nonfiction)|synthetic geometry]]. | ||
Analytic geometry is widely used in [[Physics (nonfiction)|physics]] and [[Engineering (nonfiction)|engineering]], and also in aviation, rocketry, space science, and spaceflight. It is the foundation of most modern fields of [[Geometry (nonfiction)|geometry]], including algebraic, differential, | Analytic geometry is widely used in [[Physics (nonfiction)|physics]] and [[Engineering (nonfiction)|engineering]], and also in aviation, rocketry, space science, and spaceflight. It is the foundation of most modern fields of [[Geometry (nonfiction)|geometry]], including [[Algebraic geometry (nonfiction)|algebraic]], [[Differential geometry (nonfiction)|differential]], [[Discrete geometry (nonfiction)|discrete]], and [[Computational geometry (nonfiction)|computational geometry]]. | ||
Usually the [[Cartesian coordinate system (nonfiction)|Cartesian coordinate system]] is applied to manipulate equations for [[Plane (nonfiction)|planes]], [[Line (nonfiction)|straight lines]], and [[Square (nonfiction)|squares]], often in two and sometimes in three dimensions. Geometrically, one studies the [[Two-dimensional space (nonfiction)|Euclidean plane (two dimensions)]] and [[Euclidean space (nonfiction)|Euclidean space]] ([[Three-dimensional space (nonfiction)|three dimensions]]). As taught in school books, analytic geometry can be explained more simply: it is concerned with defining and representing geometrical shapes in a numerical way and extracting numerical information from shapes' numerical definitions and representations. That the algebra of the [[Real number (nonfiction)|real numbers]] can be employed to yield results about the linear continuum of geometry relies on the [[Cantor–Dedekind axiom (nonfiction)|Cantor–Dedekind axiom]]. | |||
== In the News == | == In the News == | ||
<gallery> | <gallery> | ||
</gallery> | </gallery> | ||
| Line 25: | Line 23: | ||
* [[Geometry (nonfiction)]] | * [[Geometry (nonfiction)]] | ||
* [[Mathematics (nonfiction)]] | * [[Mathematics (nonfiction)]] | ||
* [[Synthetic geometry (nonfiction)]] - the study of Geometry using the axiomatic method and the tools directly related to them (compass and straightedge) to draw conclusions and solve problems. | |||
External links: | External links: | ||
Latest revision as of 11:38, 5 January 2019
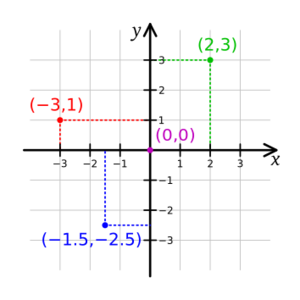
In classical mathematics, analytic geometry, also known as coordinate geometry or Cartesian geometry, is the study of geometry using a coordinate system. This contrasts with synthetic geometry.
Analytic geometry is widely used in physics and engineering, and also in aviation, rocketry, space science, and spaceflight. It is the foundation of most modern fields of geometry, including algebraic, differential, discrete, and computational geometry.
Usually the Cartesian coordinate system is applied to manipulate equations for planes, straight lines, and squares, often in two and sometimes in three dimensions. Geometrically, one studies the Euclidean plane (two dimensions) and Euclidean space (three dimensions). As taught in school books, analytic geometry can be explained more simply: it is concerned with defining and representing geometrical shapes in a numerical way and extracting numerical information from shapes' numerical definitions and representations. That the algebra of the real numbers can be employed to yield results about the linear continuum of geometry relies on the Cantor–Dedekind axiom.
In the News
Fiction cross-reference
- Crimes against mathematical constants
- Forbidden Ratio
- Geometry solvent
- Gnomon algorithm
- Gnotilus
- Mathematics
Nonfiction cross-reference
- Geometry (nonfiction)
- Mathematics (nonfiction)
- Synthetic geometry (nonfiction) - the study of Geometry using the axiomatic method and the tools directly related to them (compass and straightedge) to draw conclusions and solve problems.
External links:
- Analytic Geometry @ Wikipedia